Frank Morgan

December 2, 1999

FERMAT’S LAST THEOREM. Alf van der Poorten has written a brilliantly insightful and humorous Notes on Fermat’s Last Theorem(Wiley, 1996). Included (page 75) is the world’s shortest heuristic derivation of the Prime Number Theorem, that the number of primes less than x is asymptotic to x/log x. Later (page 89) he defines calculus as “the acknowledgment that anything but a linear function is far too complicated to handle. Differentiation provides the techniques to tame functions by making them locally linear, and integration comprises the rules for sticking the local pieces together again.”

LAST TOTALLY ODD DATE. D.D. Trent observes that “2/2/2000 will be the first time since 8/28/888 that all the digits in the date are even. That is a span of 1111 years + 158 days. Meanwhile, 11/19/1999 was the last time until 1/1/3111 that all the digits in the date are odd. That is a span of 1111 years + 43 days. That was the last totally odd date in our lifetimes.”

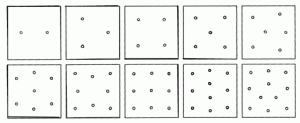
OLD CHALLENGE. Where do you think you should you place two points in a unit square to minimize the average distance in the square to the nearest of the two points? (What about more than two points?)

ANSWER. Computer calculations by Al Zimmermann yield the following configurations of 2-11 points:

Such points might provide good locations in a square country for centers of production or distribution, flour mills or computer stores. Zimmermann started with random configurations and let them evolve and improve. As yet no one has proved any of them best. Williams economist Roger Bolton and I recently have proved that for a large number of points, the ideal is approached by the vertices of a tiling by equilateral triangles, as the last two examples in the figure begin to suggest.

NEW CHALLENGE (Justin Smith). How fast do you get home with a random walk on the line? in the plane? in three-space? in n-space?

Send answers, comments, and new questions by email to [email protected], to be eligible for Flatland and other book awards. Winning answers will appear in the next Math Chat. Math Chat appears on the first and third Thursdays of each month. Prof. Morgan’s homepage is at www.williams.edu/Mathematics/fmorgan. Prof. Morgan has just been elected to the Nominating Committee of the American Mathematical Society and would welcome suggestions on members to nominate for service.

Copyright 1999, Frank Morgan.