Frank Morgan

July 1, 1999

A proof of the full Taniyama-Shimura conjecture, partly included in Wiles’s 1994 proof of Fermat’s Last Theorem, was announced last week at a conference in Park City, Utah, by Christophe Breuil, Brian Conrad, Fred Diamond, and Richard Taylor, building on the earlier work of Wiles and Taylor. The result remains tentative until the paper is written up carefully.

SHOR AND WEEKS WIN MACARTHUR AWARDS. Winners of the thirty-two MacArthur Foundation “genius awards” announced last week include computer scientist Peter Shor and mathematician Jeffrey R. Weeks. Each receives about $300,000 over five years to pursue his own interests. Shor, at AT&T Research Labs, has shown how the effect of quantum mechanics at small scales could theoretically enable a computer to quickly solve problems that would otherwise take longer than the age of the universe. Weeks, unaffiliated with any university or business, is perhaps the most notable free-lance mathematician working today. His computer program SNAPPEA will be used on data from the 2001 Microwave Anisotropy Probe (MAP) satellite to help cosmologists figure out the shape of the universe.

LEIBNIZ AND FOLDING TRIANGLES. John Sullivan observes that even Leibniz, inventor of the calculus, originally made the mistake addressed in the June 4 Math Chat on WHY IT’S HARD TO FOLD A TRIANGLE IN HALF. Leibniz wrongly thought that any fold though the center of a triangle would divide the area in half and wrote of a conversation with Huygens:

. . . I thought that a straight line drawn through the center of gravity always cut a figure into two equal parts; since that clearly happened in the case of a square, or a circle, an ellipse, and other figures that have a center of magnitude, I imagined that it was the same for all other figures. Huygens laughed when he heard this, and told me that nothing was further from the truth.

(C. H. Edwards, Jr., The Historical Development of the Calculus, Springer, 1979, p 239.)

OLD CHALLENGE. Neville Barnard likes the equation 2 + 2 = 2 x 2, and asks for other similar equations, involving two or more numbers on each side of the equation.

WINNING ANSWER (Joseph DeVincentis):

3 + 1.5 = 3 x 1.5

p + p/(p – 1) = p x p/(p – 1)

1 + 2 + 3 = 1 x 2 x 3

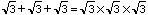
Eric Brahinsky, in a very thorough analysis, also provides some using exponentials:

2 + 2 = 2²

.346323362 + .346323362 = .346323362^.346323362 (approximately)

(the only two numbers that work like this?)

NEW CHALLENGE (Joe Shipman). Abel writes down two distinct positive integers from 1 to 4. Beta sees one of them at random and tries to guess whether it is the larger of the two. What are Beta’s chances? (What if Abel can choose from more numbers?)

Send answers, comments, and new questions by email to [email protected], to be eligible for Flatland and other book awards. Winning answers will appear in the next Math Chat. Math Chat appears on the first and third Thursdays of each month. Prof. Morgan’s homepage is at www.williams.edu/Mathematics/fmorgan. Prof. Morgan was just elected as Second Vice-President of the Mathematical Association of America.

Copyright 1999, Frank Morgan.