Frank Morgan

October 7, 1999

OLD CHALLENGE (Colin Adams). A web comment claims that, “If the population of China walked past you in single file, the line would never end because of the rate of reproduction.” Is this true?

ANSWER. Probably not, as best explained by Richard Ritter. The current population of China is about 1.25 billion, with about 20 million births per year. We’ll assume that the birthrate stays about the same, as the population grows a bit but the births per 1000 drops a bit, under the current one child per family policy. The Chinese walk say 3 feet apart at 3 miles per hour, for a rate of 46 million Chinese per year. So even if no one died in line, the line would shorten by 26 million per year and run out in about 1250/26 = 48 years. (Different assumptions could lead to a different conclusion.)

Incidentally, the UN Population Fund projects that the world population will hit 6 billion next week (around October 12).

NEW CHALLENGE with $200 PRIZE for best complete solution (otherwise usual book award for best attempt). A double bubble is three circular arcs meeting at 120 degrees, as in the third figure.

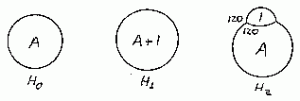
Consider a circle of area A, a circle of area A+1, and a double bubble of areas A and 1. Let H₀, H₁, H₂ denote the curvatures (1/radius) of the bottom of each. Prove that

Prove the same result for Rⁿ (replacing area by volume and circles by spheres).

This open problem appears as Conjecture 4.10 in “Component bounds for area-minimizing double bubbles,” by Cory Heilmann, Yvonne Lai, Ben Reichardt, and Anita Spielman (NSF “SMALL” undergraduate research Geometry Group report, Williams College, 1999). It bears on proving the Double Bubble Conjecture (see Math Chat of October 25, 1996).

Any individual or group is welcome to submit a solution for receipt by October 31, 1999 to Prof. Frank Morgan, Department of Mathematics, Williams College, Williamstown, MA 01267. Even incomplete solutions may compete for the usual book award.

To allow extra time for this special prize challenge, the next Math Chat will appear on November 4. Math Chat regularly appears on the first and third Thursdays of each month. Prof. Morgan’s homepage is at www.williams.edu/Mathematics/fmorgan.
[email protected].

Copyright 1999, Frank Morgan.